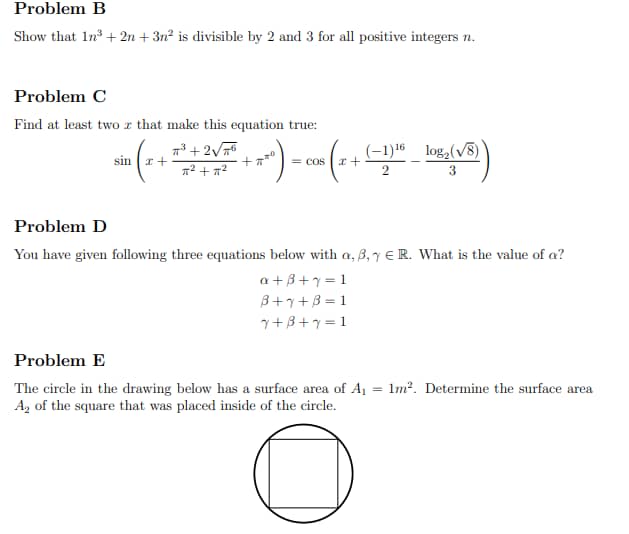
How many real roots?

Question

How many real roots does the following equation have:

x^5 + 7*x^3 + 3*x + 2 = 0.

a)0
b)1
c)3
d)5

How to solve this kind of question ?

solution in my book :

f(x) = x^5 + 7*x^3 + 3*x + 2
First derivative of f(x) is 5x^4 + 21x^2 + 3 > 0
=> f(x) is strictly increasing

f(0) = 2, f(-1) is negative
=> we have one root in (-1, 0)

I am not satisfied with this solution.
because, i don’t understand this solution.

Answer

The idea is Descartes Rule of Signs.

The mathematician Descartes ( who invented coordinate geometry by the way ), introduced a “technique” by which one can possibly tell how many real roots to expect.

Its best illustrated by an example.

Say Use Descartes’ Rule of Signs to determine the number of real zeroes of:

f (x) = x^5 – x^4 + 3x^3 + 9x^2 – x + 5.

For positive zeroes ( roots ) of f(x).

There are four sign changes. This number “four” is the maximum possible number of positive zeroes (x-intercepts) for the polynomial f (x) = x5 – x4 + 3×3 + 9×2 – x + 5. However, remember that some of the roots may be generated by the Quadratic Formula, and these pairs of roots may be complex. Then it may be that certain pairs of roots are not real, and therefore are not graphable as x-intercepts. Because of this possibility, you have to count down by two’s. That is, while there may be as many as four real zeroes, there might also be only two, and there might also be zero (none at all).

For negative zeroes, you need to replace f(x) by f(-x).

f (–x) = (–x)5 – (–x)4 + 3(–x)3 + 9(–x)2 – (–x) + 5

f (–x) = –x5 – x4 – 3×3 + 9×2 + x + 5

There is only one sign change, so there is exactly one negative root. In this case, you don’t count down by two’s, because you would end up with a negative number.

So the possibilities are :
There are 4, 2, or 0 positive roots, and exactly 1 negative root.

OK. Thats basic background work.

Now, for your question, there is no positive real roots for there is no sign changes.

f( – x ) = (-x)^5 + 7*(-x)^3 + 3*(-x) + 2
= -x^5 – 7*x^3 – 3*x + 2

So, there is one “sign” change. From [- 3*x ] to [ + 2 ].

Hence, we can conclude that the given equation has exactly one negative root.

To enumerate this root, is an altogether different matter.

ALTERNATE SOLUTION.( following your book )

The solution above is if you don’t know calculus.

With calculus,

f(x) is continuous
f(x) is strictly increasing since the book showed
f'(x) > 0 for all real x.

f(0) = + 2 is positive
f(-1)= – 9 is negative

Hence,
=> we have one root in (-1, 0)
Why? Because think about it. This kind of deduction is only true if f(x) is continuous. Continuity means that :
when x = -1, f = -9 . So starting from a negative value, as x increases (from -1 to 0) , x = 0, f = 2, means that f has “increased from” -9 to 2. Now, by continuity, as f increased from -9 to 2, at some point say x = y, f MUST have “assumed” the value 0!. That is f(y)=0 because 0 lies between -9 and 2. And this y is described by “x increases (from -1 to 0)” and thus -1 =< y =< 0, or y falls in the interval (-1,0).

We have established the “existence” of y such that
f(y) = 0 and -1 =< y =< 0.

How do we know they aren’t any other roots?
By the fact that f(x) is Increasing!
Why? Increasing means:
as x increases f(x) increases
or equivalently,
as x decreases f(x) decreases

Since there is only one root in -1 =< y =< 0, we need to consider and show NO roots for f(x) in x < -1 and x > 0.

So, for x < -1,
Increasing means (proved using calculus),
“as x decreases f(x) decreases”
So, f(x) < f(-1) = – 9 < 0.
Thus for x < -1, always f(x) < 0.
So f(x) cannot equal zero at all, implying no root here.

So, for x > 0,
Increasing means (proved using calculus),
“as x increases f(x) increases”
So, f(x) > f(0) = 2 > 0.
Thus for x > 0, always f(x) > 0.
So f(x) cannot equal zero at all, implying no root here too.

Conclusion is the same as before.
y is the real root. Only one real root.

And that’s what the book assumed obvious.

If you wanted to know the nature of the roots (not just real roots),
The answer is 1 real root and 4 complex roots.

I showed you both ways… with & without calculus.