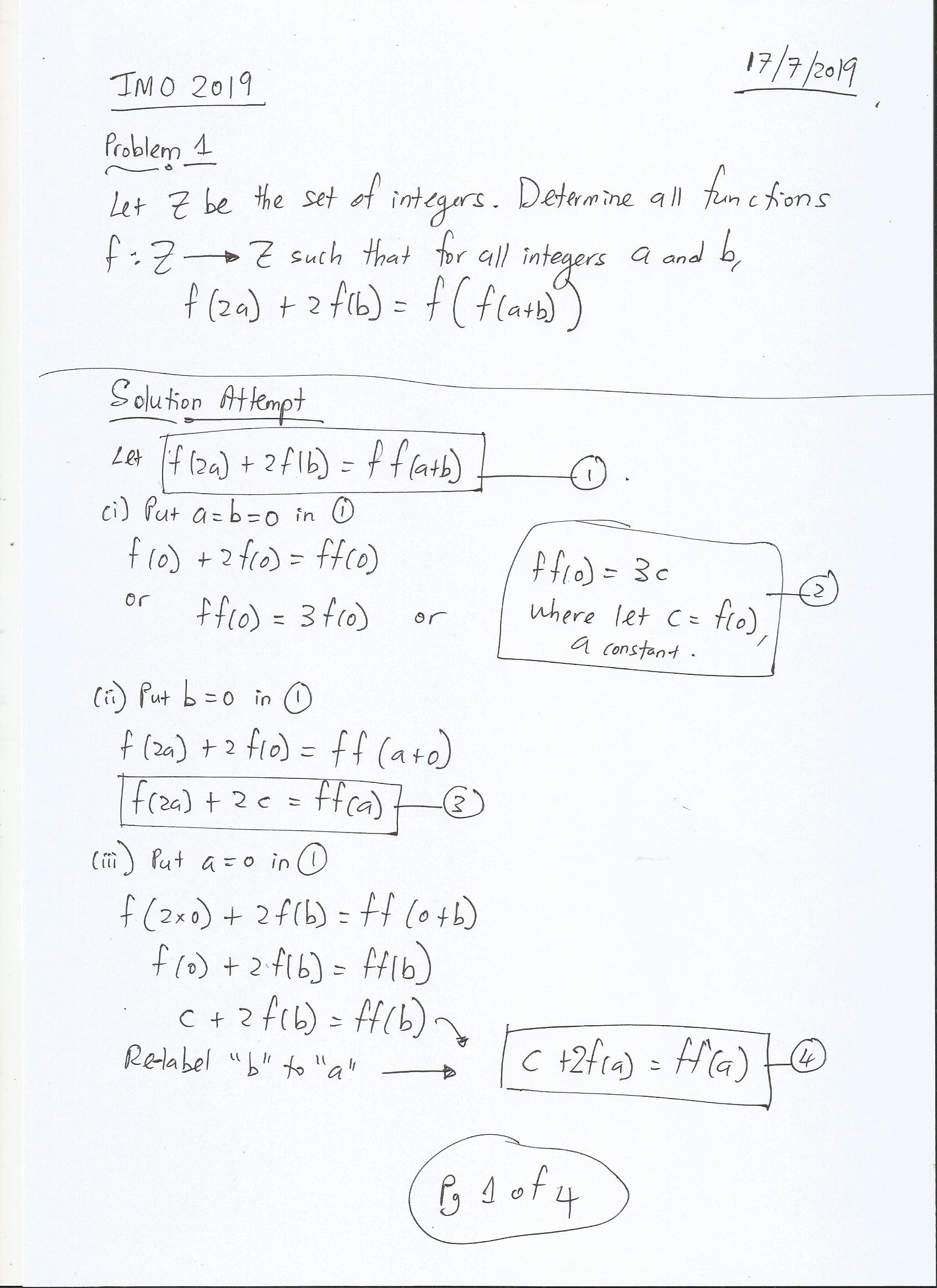
International Mathematical Olympiad IMO 2019 Problem 1

Please find the Questions for Day 1 below (Thank you Mr A for sharing it):

 

 

 

 

 

 

 

Here’s a frail attempt of mine to try solve Problem 1 to celebrate this remarkable Mathematical Competition.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

This argument may probably add rigor:

 

The fact that ff(x) is defined in definition itself means that the domain of x is the same as the codomain of f(x) proving surjective function for f and the proof is complete.

 

Why is the domain and codomain equal?

 

let

 

f(a) = ff(x)

 

So,

 

a = f(x)

 

Where

 

a = domain

 

While

 

f(x) = Codomain

 

Thus for each integer a since f(a) is defined by definition, and so is ff(x) , the function may safely be assumed to be surjective, that is for each a exists a f(x).

 

I do admit this sounds like a pseudo argument based on Convention Writing and that’s why I presented the proof as above.

 

Here’s another way to say it:

 

Yes, the last equation

 

ff(x) = 2 f(x) + c with m = f(x)

 

Causing

 

f(m) = 2m + c

 

Means that

 

m = domain because what is writable as f(m) causes m = domain by definition.

 

Hence, f(x) = m = domain due to the possible writing above as a consequence of the given functional equation.

 

But by definition

 

f(x) = Codomain

 

Thus

 

m = f(x) = domain = codomain

 

As claimed.

 

QED

 

 

 

 

 

 

 

 

 

 

 

 

Regardless whether we are able to solve these Beautiful Mathematical Problems, it does not matter in the Spiritual Context. In this Context, please be particularly careful of the ‘Pride of Life’ which comes as a natural temptation each time we make progress toward such worldly knowledge.